Processing math: 100%
Back to the class
Homework 6 (MATH 1199 Fall 2019)
1. What does the circle |z−4|=2 map to through the Mobius transformation f(z)=1z?
2. What does the circle |z−9|=3 map to through the Mobius transformation f(z)=1z?
3. Find a Mobius transformation that maps...
(a) 6↦0, −i↦1, and 64−13i↦∞
(b) −1+i↦1+i, ∞↦11, and 7+3i↦−99i
4. Approximately where are the zeros and "blow up points" in the function whose domain coloring is given in the following image?

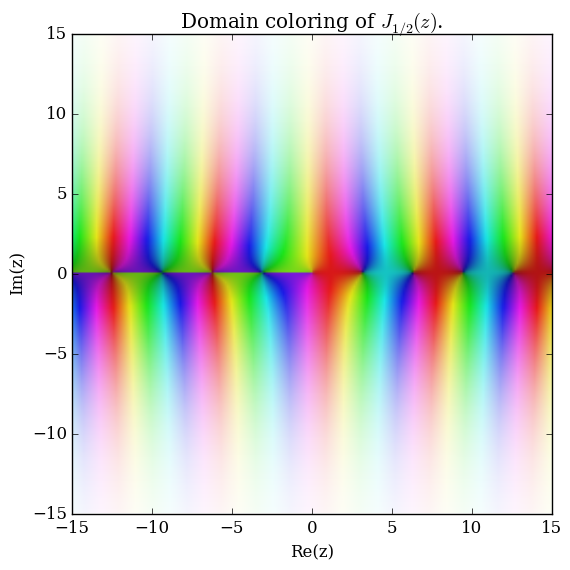
5. Approximately where is the branch cut and where are any zeros and the "blow up points" are for the function (a "Bessel function of the first kind") domain coloring is given?
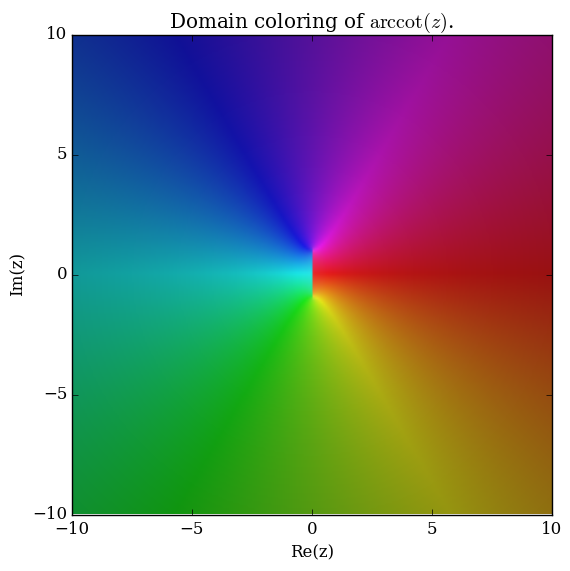
6. Approximately where is the branch cut for the inverse cotangent function pictured below? Where do you expect to find positive and negative values of this function?