Back to the class
Section 9.3 #6: If $\cos(x)=\dfrac{2}{3}$ and $x$ is in quadrant I, find $\sin(2x), \cos(2x)$, and $\tan(2x)$.
Solution: First draw this triangle so that we may find $\sin(x)$ (which will be positive because $x$ is in QI):
 Find the missing side $?$: Pythagorean theorem tells us that $2^2+?^2=3^2$, and so $?=\sqrt{9-4}=\sqrt{5}$. Therefore $\sin(x)=\dfrac{5}{3}$. Now using the double angle identity for sine,
$$\sin(2x)=2\sin(x)\cos(x)=2 \left( \dfrac{5}{3} \right) \left( \dfrac{2}{3} \right) = \dfrac{20}{9}.$$
Using a double angle identity for cosine,
$$\cos(2x)=\cos^2(x)-\sin^2(x)=\left( \dfrac{2}{3} \right)^2 - \left( \dfrac{5}{3} \right)^2 = \dfrac{4-25}{9} = -\dfrac{21}{9} = -\dfrac{7}{9}.$$
Find the missing side $?$: Pythagorean theorem tells us that $2^2+?^2=3^2$, and so $?=\sqrt{9-4}=\sqrt{5}$. Therefore $\sin(x)=\dfrac{5}{3}$. Now using the double angle identity for sine,
$$\sin(2x)=2\sin(x)\cos(x)=2 \left( \dfrac{5}{3} \right) \left( \dfrac{2}{3} \right) = \dfrac{20}{9}.$$
Using a double angle identity for cosine,
$$\cos(2x)=\cos^2(x)-\sin^2(x)=\left( \dfrac{2}{3} \right)^2 - \left( \dfrac{5}{3} \right)^2 = \dfrac{4-25}{9} = -\dfrac{21}{9} = -\dfrac{7}{9}.$$
Section 9.3 #12: Simplify
$$2\sin \left( \dfrac{\pi}{4} \right) 2 \cos \left( \dfrac{\pi}{4} \right).$$
Solution: Since $\sin \left( \dfrac{\pi}{4} \right)=\cos \left( \dfrac{\pi}{4} \right)=\dfrac{\sqrt{2}}{2}$, we calculate
$$2\sin \left( \dfrac{\pi}{4} \right) 2 \cos \left( \dfrac{\pi}{4} \right) = 2 \left( \dfrac{\sqrt{2}}{2} \right) \left( \dfrac{\sqrt{2}}{2} \right) = \dfrac{4}{4} = 1.$$
Section 9.3 #16: Find the exact value using a half angle formula:
$$\cos \left( \dfrac{7\pi}{8} \right).$$
Solution: Recall the half-angle formula for cosine states
$$\cos \left( \dfrac{\alpha}{2} \right) = \pm \sqrt{ \dfrac{1+\cos(\alpha)}{2} }.$$
Noticing that $\dfrac{7\pi}{8}$ is in quadrant II, writing $\alpha = \dfrac{1}{2} \left( \dfrac{7\pi}{4} \right) = \dfrac{\frac{7\pi}{4}}{2}$, and using the half-angle formula for cosine and the fact that $\cos \left( \dfrac{7\pi}{4} \right)=\dfrac{\sqrt{2}}{2}$ to calculate
$$\cos \left( \dfrac{7\pi}{8} \right) = -\cos \left( \dfrac{\frac{7\pi}{4}}{2} \right) = +\sqrt{\dfrac{1+\cos(\frac{7\pi}{4})}{2}} = \sqrt{\dfrac{1+\frac{\sqrt{2}}{2}}{2}}=-\dfrac{\sqrt{2+\sqrt{2}}}{2}.$$
Section 9.3 #20 If $\tan(x) = -\dfrac{4}{3}$ and $x$ is in quadrant IV, find $\sin \left( \dfrac{x}{2} \right), \cos \left( \dfrac{x}{2} \right)$, and $\tan \left( \dfrac{x}{2} \right)$.
Solution: Draw a triangle for this to get
 Find the missing side using the Pythagorean theorem: $3^2+4^2=?^2$, so $?=\sqrt{9+16}=\sqrt{25}=5$. Note that if $x$ is in QIV (with a positive rotation), then $x$ is in QII. Thus $\cos(x)=-\dfrac{3}{5}$ and $\sin(x)=\dfrac{4}{5}$. Therefore using the half angle identitites,
$$\begin{array}{ll}
\sin \left( \dfrac{x}{2} \right) &= \sqrt{\dfrac{1-\cos(x)}{2}} \\
&= \sqrt{ \dfrac{1-(-\frac{3}{5})}{2}} \\
&= \sqrt{ \dfrac{1+\frac{3}{5}}{2} },
\end{array}$$
and
$$\begin{array}{ll}
\cos \left( \dfrac{x}{2} \right) &= - \sqrt{ \dfrac{1+\cos(x)}{2} } \\
&= - \sqrt{ \dfrac{1+(-\frac{3}{5})}{2} } \\
&= - \sqrt{ \dfrac{1-\frac{3}{5}}{2} }.
\end{array}$$
Finally, we see
$$\tan \left( \dfrac{x}{2} \right) = \dfrac{\sin(\frac{x}{2})}{\cos(\frac{x}{2})} = - \dfrac{\sqrt{\frac{1+\frac{3}{5}}{2}} }{\sqrt{\frac{1-\frac{3}{5}}{2}}}.$$
Find the missing side using the Pythagorean theorem: $3^2+4^2=?^2$, so $?=\sqrt{9+16}=\sqrt{25}=5$. Note that if $x$ is in QIV (with a positive rotation), then $x$ is in QII. Thus $\cos(x)=-\dfrac{3}{5}$ and $\sin(x)=\dfrac{4}{5}$. Therefore using the half angle identitites,
$$\begin{array}{ll}
\sin \left( \dfrac{x}{2} \right) &= \sqrt{\dfrac{1-\cos(x)}{2}} \\
&= \sqrt{ \dfrac{1-(-\frac{3}{5})}{2}} \\
&= \sqrt{ \dfrac{1+\frac{3}{5}}{2} },
\end{array}$$
and
$$\begin{array}{ll}
\cos \left( \dfrac{x}{2} \right) &= - \sqrt{ \dfrac{1+\cos(x)}{2} } \\
&= - \sqrt{ \dfrac{1+(-\frac{3}{5})}{2} } \\
&= - \sqrt{ \dfrac{1-\frac{3}{5}}{2} }.
\end{array}$$
Finally, we see
$$\tan \left( \dfrac{x}{2} \right) = \dfrac{\sin(\frac{x}{2})}{\cos(\frac{x}{2})} = - \dfrac{\sqrt{\frac{1+\frac{3}{5}}{2}} }{\sqrt{\frac{1-\frac{3}{5}}{2}}}.$$
Section 9.3 #27 Calculate $\sin \left( \dfrac{\alpha}{2} \right), \cos \left( \dfrac{\alpha}{2} \right),$ and $\tan \left( \dfrac{\alpha}{2} \right)$ based on the following picture:
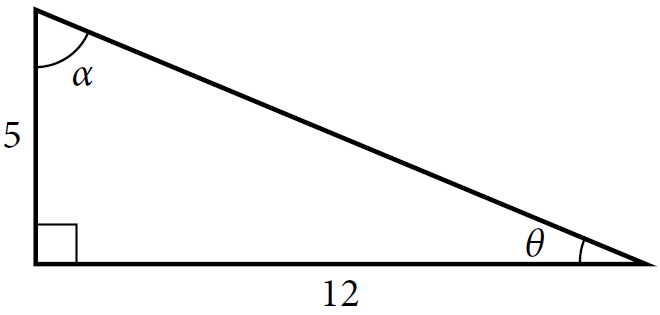
Solution: Find the hypotenuse using the Pythagorean theorem: $12^2+5^2=\mathrm{hypotenuse}^2$ and so
$$\mathrm{hypotenuse}=\sqrt{144+25}=\sqrt{169}=13.$$
In the picture, the angles $\theta$ and $\alpha$ must lie in QI and so $\dfrac{\alpha}{2}$ also lies in QI. Using the half-angle identities,
$$\sin \left( \dfrac{\alpha}{2} \right) = \sqrt{\dfrac{1-\cos(\alpha)}{2}}=\sqrt{\dfrac{1-\frac{12}{13}}{2}},$$
and
$$\cos \left( \dfrac{\alpha}{2} \right) = \sqrt{\dfrac{1+\cos(\alpha)}{2}}=\sqrt{\dfrac{1+\frac{5}{13}}{2}}.$$
Finally, we may compute
$$\tan \left( \dfrac{\alpha}{2} \right) = \dfrac{\sin(\frac{\alpha}{2})}{\cos(\frac{\alpha}{2})}=\dfrac{\sqrt{\frac{1-\frac{5}{13}}{2}}}{\sqrt{\frac{1+\frac{5}{13}}{2}}}.$$
Section 9.3 #32: Simplify the expression (do not evaluate):
$$4\sin(8x)\cos(8x).$$
Solution: This expression resembles the double angle identity for sine, $\sin(2\theta) = 2\cos(\theta)\sin(\theta)$, with $\theta=8x$. There is an extra factor of $2$ in the front (because of the $4$). So consider
$$4\sin(8x)\cos(8x)=2(2\sin(\theta)\cos(\theta))=2\sin(2\theta)=2\sin(16x).$$
Section 9.3 #39: (Use a reduction formula to) rewrite the expression with an exponent no higher than 1:
$$\cos^2(6x).$$
Solution: Using the reduction identity
$$\cos^2(\theta) = \dfrac{1+\cos(2\theta)}{2}$$
with $\theta=6x$ to get
$$\cos^2(6x) = \dfrac{1+\cos(2(6x))}{2}=\dfrac{1+\cos(12x)}{2}.$$
Section 9.3 #56: Prove the identity
$$\cos(2\alpha) = \dfrac{1-\tan^2(\alpha)}{1+\tan^2(\alpha)}.$$
Solution: The right-hand side appears (to me) to be more complicated, so I start with the right: recall the double angle identity for cosine $\cos(2\alpha)=\cos^2(\alpha)-\sin^2(\alpha)$ and compute
$$\begin{array}{ll}
\dfrac{1-\tan^2(\alpha)}{1+\tan^2(\alpha)} &= \dfrac{1-\frac{\sin^2(\alpha)}{\cos^2(\alpha)}}{1+\frac{\sin^2(\alpha)}{\cos^2(\alpha)}} \\
&= \dfrac{\frac{\cos^2(\alpha)-\sin^2(\alpha)}{\cos^2(\alpha)}}{\frac{\cos^2(\alpha)+\sin^2(\alpha)}{\cos^2(\alpha)}} \\
&= \left( \dfrac{\cos^2(\alpha)-\sin^2(\alpha)}{\cos^2(\alpha)} \right) \left( \dfrac{\cos^2(\alpha)}{\cos^2(\alpha)+\sin^2(\alpha)} \right) \\
&= \left( \dfrac{\cos(2\alpha)}{1} \right) \left( \dfrac{1}{1} \right) \\
&= \cos(2\alpha),
\end{array}$$
completing the proof.





