Back to the class
Section 3.5 #30) Sketch a graph of $y=3+\sqrt{t+2}$.
Solution: Notice that there is a horizontal shift (left by $2$) and a vertical shift (up by $3$) applied to the basic graph of $y=\sqrt{t}$. Therefore we get

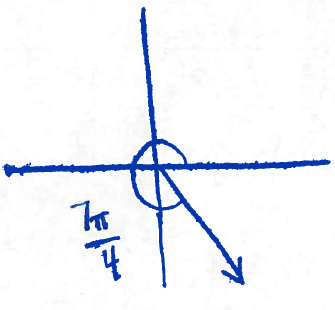
Section 7.1 #12) Draw an angle in standard position with measure $\dfrac{7\pi}{4}$ (radians).
Solution: This angle must lie in quadrant $4$ because $\dfrac{7\pi}{4}$ is bigger than $\dfrac{3\pi}{2}=\dfrac{6\pi}{4}$. Therefore we draw
Section 7.1 #24) Find the arc length indicated in the following image:

Solution: Recall the fundamental relationship: $s=r\theta$, where $s$ denotes arc length, $r$ denotes the radius, and $\theta$ the (radian) measure of the angle. In this problem, we are told $r=4.5$ and $\theta=\dfrac{2\pi}{5}$ and are asked to find $s$. Therefore
$$s = (4.5)\dfrac{2\pi}{5} = \dfrac{9\pi}{5} \approx 5.65 \mathrm{cm}.$$
Section 7.1 #27) Convert to degree measure: $\dfrac{\pi}{9}$ radians.
Solution: Recall that $180^{\circ}=\pi \hspace{2pt} \mathrm{radians}$ so that $\dfrac{180^{\circ}}{\pi \hspace{2pt} \mathrm{radians}} = 1$. Therefore compute
$$\begin{array}{ll}
\dfrac{\pi}{9} \hspace{2pt} \mathrm{radians} &= \left( \dfrac{\pi}{9} \hspace{2pt} \mathrm{radians} \right) (1) \\
&= \left( \dfrac{\pi}{9} \hspace{2pt} \mathrm{radians} \right) \left( \dfrac{180^{\circ}}{\pi \hspace{2pt} \mathrm{radians}} \right) \\
&= \left( \dfrac{180}{9} \right)^{\circ} \\
&= 20^{\circ}.
\end{array}$$
Section 7.1 #34) Convert to radians: $100^{\circ}$.
Solution:Recall that $180^{\circ}=\pi \hspace{2pt} \mathrm{radians}$ so that $\dfrac{\pi \hspace{2pt} \mathrm{radians}}{180^{\circ}}=1$. Therefore compute
$$\begin{array}{ll}
100^{\circ} &= \left( 100^{\circ} \right) (1) \\
&= \left( 100^{\circ} \right) \left( \dfrac{\pi \hspace{2pt} \mathrm{radians}}{180^{\circ}} \right) \\
&= \left( \dfrac{100 \pi}{180} \right) \mathrm{radians} \\
&= \dfrac{5\pi}{9} \mathrm{radians}
\end{array}$$
Section 7.1 #43) Find the length of the arc of a circle of radius $10$ centimeters subtended by the central angle of $50^{\circ}$.
Solution: Recall the fundamental relationship $s=r\theta$ ($\theta$ must be in radians!!!!). In this problem we are told $r=10$ and $\theta=50^{\circ}$. First convert $50^{\circ}$ into radians:
$$50^{\circ} = \left( 50^{\circ} \right) \left( \dfrac{\pi \hspace{2pt} \mathrm{radians}}{180^{\circ}} \right) = \dfrac{5\pi}{18} \mathrm{radians}.$$
With these values, we see that the desired arc length, $s$, is
$$s = 10 \left( \dfrac{5\pi}{18} \right) = \dfrac{50\pi}{18} \approx 8.73.$$





