Back to the class
Quiz 13
1.) Compute $2x(x^3+x^2+5)$.
Solution: Use the distributive law to compute
$$\begin{array}{ll}
x(x^3+x^2+5) &= x(x^3) + x(x^2) + x(5) \\
&= x^4 + x^3 + 5x.
\end{array}$$
2.) Compute $(2x-1)(x^2+7)$.
Solution: Use the distributive law twice to compute
$$\begin{array}{ll}
(2x-1)(x^2+7) &= (2x-1)(x^2) + (2x-1)(7) \\
&= 2x(x^2) - 1(x^2) + (2x)(7) - 1(7) \\
&= 2x^3 - x^2 + 14x - 7.
\end{array}$$
3.) Compute $(x-1)(x^2+2x+5)$.
Solution: Use the distributive law twice to compute
$$\begin{array}{ll}
(x-1)(x^2+2x+5) &= (x-1)(x^2) + (x-1)(2x) + (x-1)(5) \\
&= x(x^2) - 1(x^2) + x(2x) - 1(2x) + x(5) - 1(5) \\
&= x^3 - x^2 + 2x^2 - 2x + 5x - 5 \\
&= x^3 + x^2 +3x - 5.
\end{array}$$
4.) Compute $(x^2+2x+1)(x^3+3x^2+1)$.
Solution: Use the distributive law twice to compute
$$\begin{array}{ll}
(x^2+2x+1)(x^3+3x^2+1) &= (x^2+2x+1)(x^3) + (x^2+2x+1)(3x^2) + (x^2+2x+1)(1) \\
&= x^5 + 2x^4 + x^3 + 3x^4 + 6x^3 + 3x^2 + x^2 + 2x + 1 \\
&= x^5 + 5x^4 + 7x^3 + 4x^2 +2x + 1.
\end{array}$$
5.) Find one polynomial in multiple variables (completely simplified) that gives the area of the following rectangle:
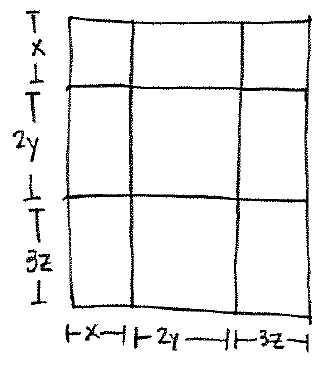
Solution: One way is to add all the areas of all the little rectangles. Another way is to realize the height of the rectangle is $(x+2y+3z)$ and the width of the rectangle is also $(x+2y+3z)$. So the area of the rectangle is (using the distributive law twice)
$$\begin{array}{ll}
\mathrm{Area} &= (x+2y+3z)(x+2y+3z) \\
&= (x+2y+3z)(x) + (x+2y+3z)(2y) + (x+2y+3z)(3z) \\
&= x^2 + 2xy + 3xz + 2xy + 4y^2 + 6zy + 3xz + 6yz + 9z^2 \\
&= x^2 + 4y^2 + 9z^2 + 4xy + 6xz + 12yz.
\end{array}$$




