Back to the class
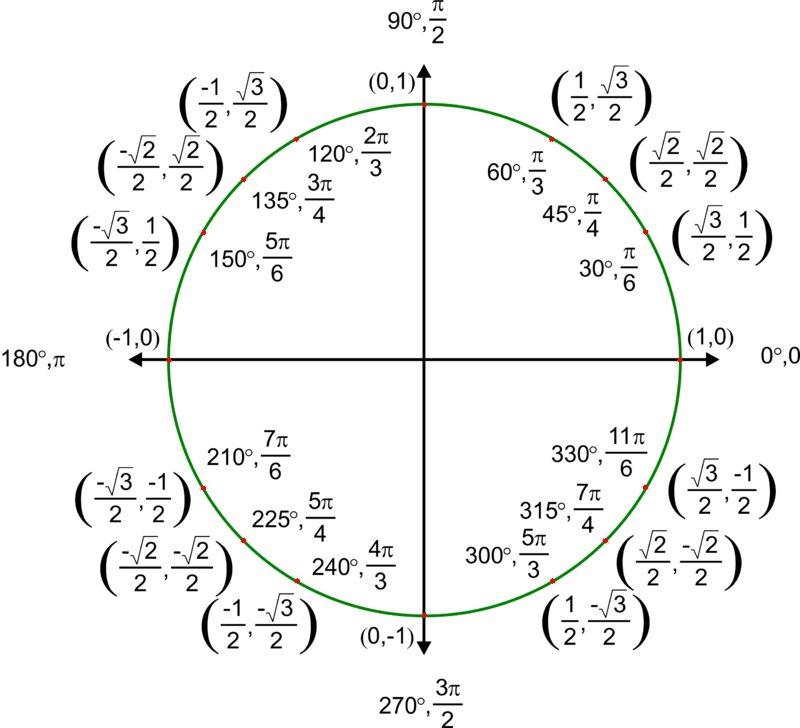
Recall the unit circle:
Section 9.5 #5: Solve on the interval $0 \leq \theta < 2\pi$: $2\sin(\theta)=\sqrt{3}$.
Solution: Isolating $\sin(\theta)$ in this expression yields $\sin(\theta) = \dfrac{\sqrt{3}}{2}$. We now look at the unit circle and find all angles whose $y$-coordinate is $\dfrac{\sqrt{3}}{2}$ and conclude that they are $\theta=\dfrac{\pi}{3}$ and $\theta=\dfrac{2\pi}{3}$.
Section 9.5 #9: Solve on the interval $0 \leq \theta < 2\pi$: $\tan(x)=1$.
Solution: We must find all angles $x$ on the unit circle whose tangent is $1$. This corresponds to the angles $x=\dfrac{\pi}{4}$ and $x=\dfrac{5\pi}{4}$.
Section 9.5 #14: Solve exactly on $[0,2\pi)$: $2\cos(\theta)=-1$.
Solution: Isolating $\cos(\theta)$ in this expression yields $\cos(\theta) = -\dfrac{1}{2}$. We must find all angles $\theta$ whose cosine is $-\dfrac{1}{2}$. The unit circle tells us this occurs at $\theta=\dfrac{2\pi}{3}$ and $\theta=\dfrac{4\pi}{3}$.
Section 9.5 #19: Solve exactly on $[0,2\pi)$: $2\cos(3\theta)=-\sqrt{2}$.
Solution: First isolate $\cos(3\theta)$ to get $\cos(3\theta) = -\dfrac{\sqrt{2}}{2}$. Since the argument to cosine is not a single symbol, we introduce a new symbol $\psi$ that is defined by $\psi=3\theta$. This means our equation becomes $\cos(\psi) = -\dfrac{\sqrt{2}}{2}$. From the unit circle, we see that the possible values that $\psi$ may take are $\psi=\dfrac{3\pi}{4}$ and $\psi=\dfrac{5\pi}{4}$. However, this is not a solution to the original equation, because we must solve for $\theta$ -- also the fact that the original function had "$3\theta$" means we may end up with more solutions. So recall we want $0 \leq \theta < 2pi$ and we have general solutions
$$3\theta = \psi = \dfrac{3\pi}{4} + 2n \pi, \quad n=\ldots,-1,0,1,\ldots$$
and
$$3\theta = \psi = \dfrac{5pi}{4} + 2n \pi, \quad n=\ldots, -1,0,1, \ldots.$$
Dividing by $3$ yields
$$\left\{ \begin{array}{ll}
\theta = \dfrac{3\pi}{12} + \dfrac{2n\pi}{3} = \dfrac{3\pi}{12} + \dfrac{8n\pi}{12} \\
\theta = \dfrac{5\pi}{12} + \dfrac{2n\pi}{3} = \dfrac{5\pi}{12} + \dfrac{8n\pi}{12}.
\end{array} \right.$$
Now we must find all values for $n$ that yield a $\theta$ that is in the range $0 \leq \theta < 2\pi$, or in other words, $0 \leq \theta < \dfrac{24\pi}{12}$. First try $n=0$:
$$n=0: \left\{ \begin{array}{ll}
\theta = \dfrac{3\pi}{12} + 0 = \dfrac{3\pi}{12} \checkmark \\
\theta = \dfrac{5\pi}{12} + 0 = \dfrac{5\pi}{12} \checkmark
\end{array} \right.$$
now try $n=-1$:
$$n=-1: \left\{ \begin{array}{ll}
\theta = \dfrac{3\pi}{12} - \dfrac{8\pi}{12} = -\dfrac{5\pi}{12} \mathbf{\times} \\
\theta = \dfrac{5\pi}{12} - \dfrac{8\pi}{12} = -\dfrac{3\pi}{12} \mathbf{\times}
\end{array} \right.$$
so the rest of the negatives will also fail. Now try $n=1$:
$$n=1: \left\{ \begin{array}{ll}
\theta = \dfrac{3\pi}{12} + \dfrac{8\pi}{12} = \dfrac{11\pi}{12} \checkmark \\
\theta = \dfrac{5\pi}{12} + \dfrac{8\pi}{12} = \dfrac{13\pi}{12} \checkmark
\end{array} \right.$$
Now try $n=2$:
$$n=2: \left\{ \begin{array}{ll}
\theta = \dfrac{3\pi}{12} + \dfrac{16\pi}{12} = \dfrac{19\pi}{12} \checkmark \\
\theta = \dfrac{5\pi}{12} + \dfrac{16\pi}{12} = \dfrac{21\pi}{12} \checkmark
\end{array} \right.$$
Now try $n=3$:
$$n=3: \left\{ \begin{array}{ll}
\theta = \dfrac{3\pi}{12} + \dfrac{24\pi}{12} = \dfrac{27\pi}{12} \mathbf{\times} \\
\theta = \dfrac{5\pi}{12} + \dfrac{24\pi}{12} = \dfrac{29\pi}{12} \mathbf{\times}.
\end{array} \right.$$
From the $n=3$ case we see that all larger $n$ will also fail. Therefore we have found 6 solutions:
$$\theta = \dfrac{3\pi}{12}, \dfrac{5\pi}{12}, \dfrac{11\pi}{12}, \dfrac{13\pi}{12}, \dfrac{19\pi}{12}, \dfrac{21\pi}{12}.$$
Section 9.5 #23: Find all solutions on $[0,2\pi)$: $\sec(x)\sin(x)-2\sin(x)=0$.
Solution: Factor out the common factor $\sin(x)$ to get the equation
$$\sin(x) (\sec(x)-2)=0.$$
Using the "zero-product property" (i.e. if $ab=0$ then $a=0$ or $b=0$) we see that this equation boils down to solving two simpler equations: $\sin(x)=0$ and $\sec(x)-2=0$. First let us solve $\sin(x)=0$: from the unit circle we may conclude that the only solutions in $[0,2\pi)$ are $\theta=0,\pi$. Now let us solve $\sec(x)-2=0$. First solve for $\sec(x)$ by adding $2$ to both sides to get $\sec(x)=2$. Recall that $\sec(x) = \dfrac{1}{\cos(x)}$ and plug that in to get $\dfrac{1}{\cos(x)}=2$. Take the reciprocal of both sides (i.e. flip both sides upside down) to get $\cos(x)=\dfrac{1}{2}$. From the unit circle we see that the solution is $x=\dfrac{\pi}{3}, \dfrac{5\pi}{3}$. Therefore we have found four solutions:
$$x=0,\pi,\dfrac{\pi}{3}, \dfrac{5\pi}{3}.$$
Section 9.5 #28: Find all solutions on $[0,2\pi)$: $\cos^2(\theta) = \dfrac{1}{2}$.
Solution: Taking the square root of both sides (do not forget you must consider both positive and negatives when taking the square root in an equation), we get $\cos(\theta) = \pm \sqrt{\dfrac{1}{2}}$. Note that the number $\sqrt{\dfrac{1}{2}}$ is just a different way to write the number $\dfrac{\sqrt{2}}{2}$ because
$$\sqrt{ \dfrac{1}{2}} = \dfrac{\sqrt{1}}{\sqrt{2}} = \dfrac{1}{\sqrt{2}} = \dfrac{1}{\sqrt{2}} \left( \dfrac{\sqrt{2}}{\sqrt{2}} \right)=\dfrac{\sqrt{2}}{2}.$$
Therefore we must find solutions to $\cos(\theta)=\dfrac{\sqrt{2}}{2}$ and to $\cos(\theta)=-\dfrac{\sqrt{2}}{2}$. The unit circle shows us that all such $\theta$ are
$$\theta=\dfrac{\pi}{4}, \dfrac{3\pi}{4}, \dfrac{5\pi}{4}, \dfrac{7\pi}{4}.$$
Section 9.5 #38: Find all solutions on $[0,2\pi)$: $\sin(2t)=\cos(t)$.
Solution: Use the double angle identity for sine on the left to write
$$2\sin(t)\cos(t) = \cos(t).$$
Subtract $\cos(t)$ from both sides to get
$$2\sin(t)\cos(t)-\cos(t)=0.$$
Factor $\cos(t)$ out to write
$$\cos(t)(2\sin(t)-1)=0.$$
Now we use the zero-product property to say $\cos(t)=0$ and $2\sin(t)-1=0$. Solving $\cos(t)=0$ yields $t=\dfrac{\pi}{2}, \dfrac{3\pi}{2}$. Solving $2\sin(t)-1=0$ for $\sin(t)$ yields $\sin(t)=\dfrac{1}{2}$ and so we solve that to get $t = \dfrac{\pi}{6}, \dfrac{5\pi}{6}$. Therefore we get the four solutions $t=\dfrac{\pi}{2}, \dfrac{3\pi}{2}, \dfrac{\pi}{6}, \dfrac{5\pi}{6}$.
Section 9.5 #94: If a loading ramp is placed next to a truck, at a height of $4$ feet, and the ramp is $15$ feet long, what angle does the ramp make with the ground?
Solution: First draw the situation:
Notice that $\sin$ relates the two known sides to the unknown angle in the following way:
$$\sin(\theta)=\dfrac{4}{15}.$$
Take the inverse sine of both sides to compute
$$\theta = \sin^{-1} \left( \dfrac{4}{15} \right) \approx 0.2699 \mathrm{\hspace{2pt} rad}.$$
An answer in terms of degrees is also ok.
Section 9.5 #96: A woman is watching a launched rocket currently 11 miles in altitude. If she is standing 4 miles from the launch pad, at what angle is she looking up from horizontal?
Solution: First draw the situation:
Notice that the $\tan$ relates the two sides to $\theta$ so we write
$$\tan(\theta) = \dfrac{11}{4}.$$
To find the angle $\theta$, take the inverse tangent of both sides to compute
$$\theta = \tan^{-1} \left( \dfrac{11}{4} \right) \approx 1.222 \mathrm{\hspace{2pt} rad}.$$
An answer in terms of degrees is also ok.
