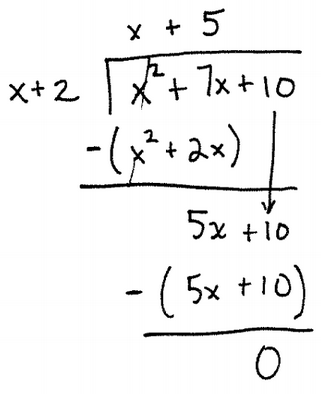Back to the class
Quiz 15
1.) Perform the long division:
$$(x^2+7x+10) \div (x+2).$$
Solution:
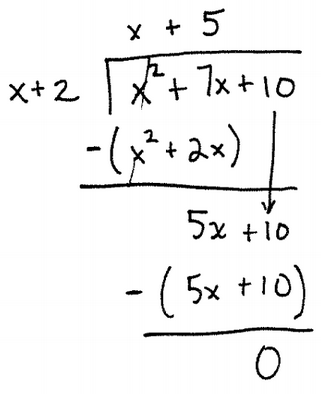
Therefore the answer is
$$(x^2 +7x+10) \div (x+2) = x+5.$$
2.) Factor by grouping:
$$6x^3 + 4x^2 + 3x + 2.$$
Solution: Calculate
$$\begin{array}{ll}
(6x^3 + 4x^2) + (3x+2) &= 2x^2 (3x+2) + (3x+2) \\
&= (2x^2+1)(3x+2).
\end{array}$$
3.) Factor or state that it is prime:
$$x^2+3x-10.$$
Solution: We seek numbers $p$ and $q$ so that $pq=-10$ and $p+q=3$. We ask how $-10$ may be factored: $-10 \cdot 1$, $10 \cdot (-1)$, $-2 \cdot 5$, or $2 \cdot (-5)$. From these we observe that the only option is the pair $-2$ and $5$ because $-2+5=3$. This means we will take $p=-2$ and $q=5$. Therefore we rewrite the original polynomial in the following way:
$$x^2+3x-10=x^2-2x+5x-10.$$
Now we factor by grouping:
$$(x^2-2x)+(5x-10) = x(x-2) + 5(x-2) = (x+5)(x-2).$$
4.) Factor or state that it is prime:
$$2x^2+x-3.$$
Solution: We seek $p$ and $q$ so that $pq=2(-3)=-6$ and $p+q=1$. Look at how $-6$ may be factored: $-6 \cdot 1$, $6 \cdot (-1)$, $3 \cdot (-2)$, or $-3 \cdot 2$. From these we observe that the only option is the pair $3$ and $-2$ because $3+(-2)=1$. This means we will take $p=3$ and $q=-2$. Therefore we rewrite the original polynomial in the following way:
$$2x^2+x-3=2x^2+3x+(-2)x-3.$$
Now we factor by grouping:
$$(2x^2+3x)+(-2x-3) = x(2x+3)+(-1)(2x+3) = (x-1)(2x+3).$$
5.) Factor or state that it is prime:
$$x^2+13x-2.$$
Solution: We seek $p$ and $q$ so that $pq=-2$ and $p+q=13$. Look at how $-2$ may be factored: $-2 \cdot 1$ or $2 \cdot (-1)$. From this we observe no pair can satisfy the requirement that $p+q=13$, and so we say that this polynomial is prime.