Back to the class
Quiz 12
1.) Simplify $\dfrac{(3x)^2(y^4)}{x(3y)^2}$.
Solution: First use the law $(ab)^n=a^nb^n$ to see $(3x)^2=9x^2$. Similarly, $(3y)^2=9y^2$. Using the law $\dfrac{a^n}{a^m}=a^{n-m}$, we see
$$\dfrac{(3x)^2(y^4)}{x(3y)^2} = \dfrac{9x^2y^4}{9xy^2}=9^0 x^{2-1} y^{4-2}=xy^2.$$
2.) Simplify $\left( \dfrac{2w^3y^2x}{3z} \right)^2 \left( \dfrac{z^2}{w^2y} \right)$.
Solution: First we use the law $\left( \dfrac{a}{b} \right)^n = \dfrac{a^n}{b^n}$ to handle the first. Then we will use the law $(ab)^n=a^n b^n$ multiple times to handle the top and bottom. After that the law $(a^n)^m=a^{nm}$ applies to begin simplifying. Then we use the law $\dfrac{a^n}{a^m}=a^{n-m}$ to finish simplifying. We get
$$\begin{array}{ll}
\left( \dfrac{2w^3y^2x}{3z} \right)^2 \left( \dfrac{z^2}{w^2 y} \right) &= \left( \dfrac{(2w^3 y^2 x)^2}{(3z)^2} \right) \left( \dfrac{z^2}{w^2y} \right) \\
&=\left( \dfrac{4w^6 y^4 x^2}{9z^2} \right) \left( \dfrac{z^2}{w^2y} \right) \\
&= \dfrac{4}{9} w^{6-2} y^{4-1} x^2 z^{2-2} \\
&= \dfrac{4}{9} w^4 y^3 x^2,
\end{array}$$
where the $z$ has disapeared because $z^{2-2}=z^0=1$.
3.) Polynomial or not?
a.) $3x^2+11x+4$
b.) $\dfrac{1}{x} + \dfrac{1}{x^2} + \dfrac{3}{x^3}$
c.) $5$
d.) $\sqrt{x} + 2x^2+1$
Solution: Leters a.) and c.) are polynomials. Letters b.) and d.) are not. Letter b.) is not because polynomials do not allow division by $x$. Letter d.) is not because it has a square root.
4.) Simplify $(3x^2+5x-7)-(x^2-2x+1)$.
Solution: Remember we must distribute the minux sign into the second term. This yields
$$(3x^2+5x-7)-(x^2-2x+1)=3x^2+5x-7-x^2+2x-1=2x^2+7x-8.$$
5.) Write the area of the big square in 2 different ways:
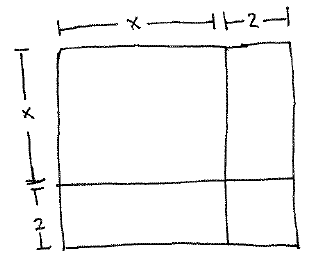
Solution: The first way will be to recognize that each side height of the rectangle is $x+2$ and the width of the rectangle is $x+2$. Since the area of any rectangle is its length multiplied by its width, the area could be written as $(x+2)(x+2)=(x+2)^2$. The second way is to write the sum of the four rectanlges inside of the big rectanlge. The top left one has area $x^2$, the top right one has area $2x$, the bottom left one has area $2x$, and the bottom right one has area $4$. Therefore the area of the whole square is $x^2+2x+2x+4=x^2+4x+4$.



